The recent curriculum renewal has placed a (well-deserved) heightened emphasis on counting, set theory, and probability. Just under a half of a Grade 12 “Foundations of Mathematics” course now covers the three topics. This is a huge improvement from the token, disjointed topics strewn around the last courses. It allows teachers to set a different tone–a tone of curiosity that seems inherent in probability.
I came across the idea of Grime Dice (named and pioneered by Dr. James Grime @jamesgrime) late last year after I knew I was to be teaching probability this winter. I knew right away this was a great task to get students tinkering with probability before defining its inter-workings theoretically. A great description of their function can be found on the PlusMath website written by Dr. Grime himself. They are available for purchase from MathGear.co.uk.
Basically, the dice have been altered to compete at different strengths against varying dice. A very interesting phenomenon all to itself. I wanted to find a way to capture the simplicity of a two-dice game, couple it with the quirkiness of non-transitivity, and wrap it all up in a task filled with student initiative. My result follows.
I began by purchasing five novelty dice from a dollar store. I re-worked the numbers on the sides to match Grime Dice A, C, and E. (see previously linked article). I didn’t make dice for every group, but the task could be extended quite neatly into an experimental probability lab.
To begin the class, I introduced the class to the altered dice by showing them a graphic.
I then handed out a sheet with three dice charts on it.
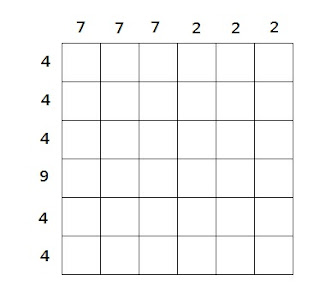 |
| Dice Chart between Grime Dice “A” and “C” |
Students were then asked to answer the question:
I circulated and probed further as groups finished the relatively easy task. Soon students began to see the circular nature of the dice. As a class, I explained how this could be exploited by choosing last in a game. We also talked about the probabilities themselves. Students had a natural grasp on odds and probability and offered great explanations for their value. We briefly touched on possible values for probabilities, and the idea of a complement.
Enter Phase Two.
I attempt to build in student authorship into tasks. Student thought flourishes when they are not only problem-solvers, but also problem-creators. The second portion of the lesson was designed to get students creating mathematics.
I posed the following problem while handing out a fresh set of three (blank) dice charts: