My province is in the midst of a major overhaul on its curriculum. This puts me in a very interesting situation. I am a new teacher in a large division filled with veteran teachers that all feel as overwhelmed as myself. I can’t decide if this is a curse or a blessing; I simply continue to roll with all the punches that curriculum renewal brings. On top of the nuts-and-bolts of each new course (5 of which I teach for the first time this year), the division heaps on division, school, department, and personal learning priorities. To make matters even more confusing, each initiative comes with about 35 acronyms. I can’t tell the difference between AFL, PLO, PLP, PPP, SLI, PBL… you get my drift. Amidst the chaos of red tape, I believe I have found something to hang my hat on.
Our department goal is to find creative ways to develop and foster a growth mindset in our students. Our school has a very large proportion of unsuccessful students. Many students feel as though math is too difficult, or genetics has blocked their possible success. I read Dr. Carol Dweck’s book Mindset last year as a part of school book clubs, and found its message intriguing. I coupled this with research I have been doing into Problem Based Learning in Mathematics. The creation of the new “Workplace and Apprenticeship Mathematics” pathway in my province completed the perfect storm of personal learning. All these factors contributed to my brainchild.
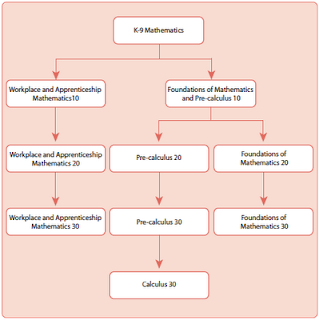
A little more explanation needs to go into the pathways in Saskatchewan. Grade 9 math is taken by every student. In Grade 10, there are 2 options–Foundations and Pre-calculus 10 and Workplace and Apprenticeship 10. In Grade 11, FPC10 splits further into 2 pathways–Foundations 20 and Pre-calculus 20. Workplace and Apprenticeship Mathematics 20 is also offered. In Grade 12, all 3 strands are offered at the 30 level along with Calculus 30. The whole idea is for students to take the mathematics that is suited for them and their future.
The Workplace and Apprenticeship pathway is designed to build skills pertinent in a work setting. The textbooks are designed around unit projects, and the lessons focus on application. Links to the Saskatchewan Curriculum are found below:
- Every student/group is given a binder with a print out of the curricular objectives in it. The binder includes rubrics, a list of sample projects, and a stock of daily log sheets.
- Students/groups will design/brainstorm projects that fit specific curricular objectives. They will create a project proposal and get it approved by me before they start.
- Each project works on highlighted areas of the curriculum. Their outcomes will be physically highlighted in their binders.
- Every day ends by filling out a daily log sheet which details who was present, what was discussed, decisions that were made, progress to report, and a plan for the future.
- Every project ends with a product. A short presentation (business style) is made to me or the class at the conclusion.
- Students are able to overlap objectives in successive projects to attain a higher grade for that outcome. The grade for that outcome is never fixed; students may always grow in their understanding of a topic.
- Evaluation of each project is based on a rubric (developed in tandem with the group). Self and group evaluation will also play a major role.
This is an early stab at my vision. I have rough outlines of the group log sheets and rubrics, but nothing is final yet. My hope is to present this framework as my attempt at developing a growth mindset in my students. It also satisfies my curiosity for PBL and creates an authentic workspace for the the workplace mathematics to unfold. There are a few problems that I have to address (and if you can think of more, please comment!)
- What do I do for students that do not show up for class?
- What about students that complete all goals before the semester ends?
- What percentage of the goals completed can be considered a pass?
- Do I simply check off each outcome, or give a letter grade for how well it was met? Possibly a rubric 0-4?
- How can I possibly control the freedom granted with group netbooks?
- Do I allow very similar projects?
- How large of a scope can a single project encompass?
- How should I handle an in-class topic that needs to be widely clarified?
Ironically, the design of this class pathway is a perfect example of Problem Based Learning for myself. My short career has not contained an idea quite like it. It requires tremendous administrative and departmental support (which I feel I could get), but also needs wide-scale fine tuning. I am hoping the twitterverse can lend me some all important critical feedback.
6 replies on “Proper Workspace for Workplace”
So pumped that you'll be teaching this Nat.
My suggestion might be to model a first problem (maybe decided by consensus?) with the class as a whole. You could still incorporate options into the project, but have the class go through the project more or less as a group. This way you can model, scaffold & support all the things you want to see with all future projects. I think this will also be a good way for you to see when it's appropriate to bring the whole group together for a quick lesson, half the class, or just a couple of students.
I think you could avoid grades all together, but that will likely depend on what kind of support you have from your admin & dept. I would set some kind of markers throughout the year where students need to have a certain # of outcomes met so you don't have a mad panic situation come end of May.
Looking froward to seeing this take shape,
@park_star
Hi Nat! I'm a middle school math teacher in Alberta that shares your vision of how a math classroom should look!
Our students need lots of support, and there are growing pains, but I believe if the tasks you and your students design are engaging "Goldilocks" tasks, then your class management and assessment issues will be ironed out as you go. Don't give up, and keep reinventing your process until it works.
testing one two three
Hi Nat,
I don't have to email you because I can post a comment when I use Google Chrome instead of IE. Anyway, I just wanted to say that I have been thinking more seriously about projects since I read Jo Boaler's research. However, in reality, my framework for math instruction is 1) relationship; 2)access; 3) mathematical discourse(equity). So I have read and absorbed a lot about projects, but I haven't really done any projects that span over many class periods. Of course, this got me to thinking…what is a project? So I put them in three categories:
1) Discover math and it's beauty by using numbers only (like investigate Pascal's triangle)
2) Integrate mathematics into a situation that students are familiar with like buying an item at the store
3) Dan Myer's projects
For various project ideas:
I belong to edweb.net which is a great math community in Northern CA, and a lot of teacher post projects or ideas similar to twitter, but it's more focused. You would be welcome to join the community. I've seen a lot of project ideas.
I know that @yummymath has projects on their website that are type #2. I'm sure you are familiar with Dan Myer's website where he uses the video camera and technology to have students drive the mathematical questions.
When I taught students in an alternative program, I created a project and a slew of worksheets based on NCTM illuminations using a 10 x 10 square to determine 1 percent of any number and then determine a percentage from the 1%. Let me know if you are interested I can email you chico 2 mom at sbc global net
Also found some great algebra projects on this blog: http://ispeakmath.wordpress.com/
I look forward to hearing what you put together. Best to you!
Lisa
p.s. these are three websites I got from edweb…I glanced at them a while ago:
http://nrich.maths.org
http://plus.maths.org/content/
http://motivate.maths.org/content/
Lisa
Hey Nat, glad you're able to "hang your hat" on PBL. We've found PBL to be a huge boon for student understanding, problem solving ability, and literacy skills.
Recently, I've started a crowd-sourced curriculum map for several content areas, aligning with a PBL curriculum. Please go here to take from and add to it:
http://emergentmath.wordpress.com/the-great-inquiry-based-math-curriculum-mapping-project/
–Geoff