My wife and I spend a lot of time with friends who have three young children. I spend most of that time engaged in a combination of trampoline dodge ball and mathematical discourse. The middle child is most willing to think mathematically. During one of our conversations, he decided to turn the tables. What resulted is a wonderful look into a child’s perception of what “mathematics” does.
Him: Maybe you can answer my question?
Me: Sure. What is it?
Him: Ummm… (literally scratches head)
I could tell that he was reaching for straws, but just as I was going to suggest some possible pathways, he began detailing his problem. What followed was a hodge-podge of textbook drivel. Roads met at points, trains travelled at speeds, the vehicles took neatly-timed breaks, and the whole thing finsished with a simple question:
Will the trains crash?
After the initial digestion of the problem, I asked him to draw it out so I could get a visual. Despite major changes in the question, he managed to draw this diagram and detail the problem:
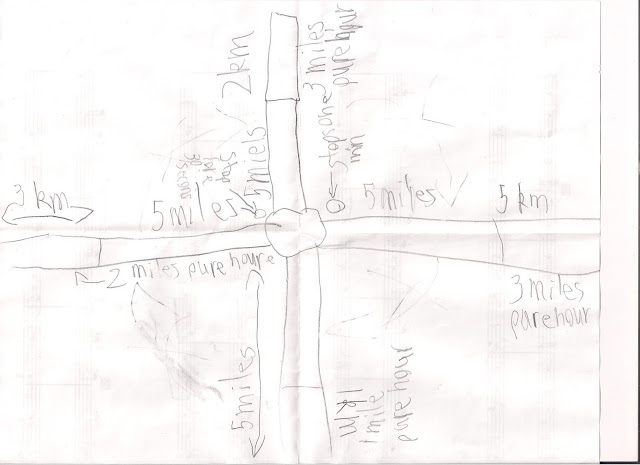 |
| His “textbook ready” diagram |
Ok… um. There are 4 trains. One of them is 3km long. Another is 2km long. Another is 5km long. Another is 1km long. The 3km train goes 2mph. The 2km train goes 3mph. The 5km train goes 3mph and the 5km train goes 3mph.
All the tracks are five miles long. The 5km train stops at a stoplight for one minute. The 3km train stops for 30 seconds. Will the trains crash?
There are so many awesome things going on here. First, we must keep in mind that the child’s natural curiosity has been polluted by school mathematics. He constantly shows desire to make sense of his world through mathematics, but when required to produce a question, he can only think of manufactured, one-size-fits-all textbook problems. He hasn’t even covered the mathematics of rates but knows that, when doing “math”, it is important to list every detail neatly and orderly. He knew that each track needed a length and each train needed a speed. He even managed to mix up his units–for good measure. (Pun intended).
Second, the child makes the problem more difficult by adding more details. When I asked him if he could make the problem easier, he told me I could take away one of the trains. To him, more details always equals more difficulty. I asked him to make the problem more difficult, he paused and then told me that an asteroid hits Train 1.
Third, he assumes that there is no real basis for the questions his teachers ask him. He knows that they include real life objects (such as trains, stoplights, and asteroids), but has no inkling that mathematics is used to model and describe real life. He also has a sense of mathematical neatness. The entire situation is tied up with one, nice question: Will the trains crash?
We have to be constantly aware of what are students are perceiving as important details. We have built an educational culture where all the details are readily available to those who are willing (or are literate enough) to search for them within drawn out paragraphs of textbook banter. Students need to be presented mathematical situations where they are required to make decisions, gather resources, and apply strategies. I’m worried that school is not offering opportunities for students to reason and model mathematically. What would your students create if asked to write a math question?
NatBanting
One reply on “Talking with Children: Word Problems”
Your blog post here really has me thinking. For 11 years, I taught Kindergarten to Grade 2, and this year, I moved up to Grade 6. Before I started, I was told by some fellow teachers that I was going to have to start using textbooks. "Junior math is taught with textbooks." I struggled with this. I never used textbooks when teaching JK-2, and I loved getting students to think deeply about math, wonder about math, and share their learning about math. I wanted this to happen in Grade 6 as well.
I spoke to the Math Facilitator at our school at the time, and she helped me see that even in Junior grades students can be taught without relying on the textbook. In fact, what I learned this year when looking at the textbook is that it`s highly outdated and does not address all current curriculum expectations. So I moved beyond the textbook.
It took a while to get students to adjust. They didn't want to "wonder" about math. They were hesitant to use manipulatives. And they were searching for that question with the one answer that was "right."
Now students are excited about math. They're eager to think about why we're learning what we're learning. They are digging deeper into math concepts, and expressing their own "wonders." They are thinking beyond the textbook problem, and I hope they'd write a more open-ended problem that goes beyond the "textbook" as well.
Thanks for getting me to reflect!
Aviva
http://www.weinspirefutures.com