Fractions, factors, and functions.
A large portion of my career to date has been spent musing over how to engineer classroom environments that infuse meaning into these three mathematical structures. When it comes to polynomial factoring, the area model has provided the most success. After connecting 2-digit by 2-digit multiplication, the area model becomes a beautiful visual to make sense of the “adds to ___; multiplies to ___” phrase that echoes around the room.
But we don’t keep the area model around forever. Once we’ve used the model to build meaning, we mobilize that understanding in more symbolic situations in a careful, deliberate march toward mathematical abstraction.
When it comes to designing these sorts of symbolic, pattern-based tasks, I see them going in three potential directions:
- Here is a polynomial, what are the factors? (Enter every single textbook ever written)
- Here are some parameters, build a polynomial that satisfies them. (Build a trinomial that has a non-1 GCF and also a factor of “x – 3” etc.)
- Meet in the middle. (Here are a set of both polynomials and factors, match them up).
After some experimentation, I discovered that the “meet in the middle” provides a curious possibility when working with polynomial factoring: Students must select factors and terms based on the factoring patterns we are aiming to solidify, but also must keep an eye on what is /not/ being chosen. That is, with every choice toward completing all the matches, the pressure is put on to leave pieces that work well together. Because of this, the sense-making amplifies as you go along. This feels like a dream scenario for the teacher.
The Task
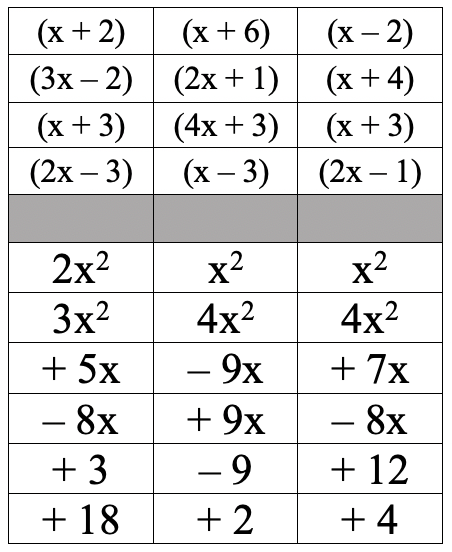
I built six trinomials for this particular matching task. There was a touch of intentional design in my choices, but nothing incredibly complex. Essentially, I was trying to create some nice starting places, but also include enough possible overlap to result in a variety of strategies. (Of course, you can add, adapt, or subtract pieces to your heart’s content). I printed off the polynomials on a single piece of cardstock and handed out scissors to each group.
Once all the pieces were cut out, the students were asked to match (and multiply) two factors from the top section with three terms from the bottom section in order to reconstruct six true trinomial multiplication statements. Each of the polynomials must be used in exactly one statement.
I also had a single example prepared to clarify the process. It didn’t involve any of the factors from this list of six, but showed how the factors are selected and multiplied and the terms from the bottom section are combined to form trinomials.
As the student groups worked, I found the following teaching prompts helpful:
- What helps you narrow down the options for pairings?
- Are there any factors that cannot be paired?
- Which numbers give you the most information?
- How could you create that “4x2” term?
- How does the addition/subtraction in the factors influence your choices?
We did this first set as a group activity shortly after employing the area model to understand the symbolic pattern. Student groups then built their own set of six polynomials to trade with a neighbouring group. It was very fun to listen to their rationale as they tried to build an interesting set.
Ultimately, this is a relatively simple idea that sets the stage for mathematical pattern-seeking and sense-making, and that’s a sweet spot I’m looking for in my classroom.
NatBanting
P.S. Against my better judgement, the resolution to the task can be found here, but (maybe) you should spend some time matching before ruining it for yourself.